| Amplitude | Day | Ex Meridian | Exercises | Pole Star | |
| Naut. Almanac |
Celestial Navigation
Dip
Dip of
horizon
We know from physics that a ray of light is bent
(refraction) when passing from a denser to a rarer medium and vice versa. On
the earth’s surface the atmosphere, which is present, is made up of several
layers of varying densities, as such a ray of light gets bent due to the above
phenomenon. If the earth’s surface were flat, visible and sensible horizon
would be identical, however the visible horizon appears several arc minutes
below the sensible horizon, which is the result of two opposing effects:
- The
curvature of the earth’s surface and
- The
atmospheric refraction.
Atmospheric refraction thus bends light rays passing
along the earth’s surface toward the earth, all points on the horizon appear to
be elevated, giving rise to a false horizon, and this
is termed VISIBLE HORIZON.
The sensible horizon is the actual horizon that the
observer would see if the atmosphere was not present or the atmosphere density
was uniform as well if the earth were flat and not round.
Thus the visible horizon is some arc minutes below the
sensible horizon.
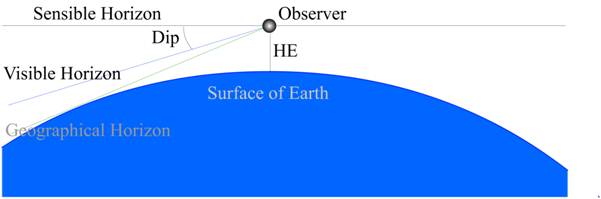
The altitude of the sensible horizon relative to the
visible horizon is called dip and is a function of the height of eye,
HE, the vertical distance of the observer’s eye from
the earth’s surface:
Dip (in minutes) = 1.76√Height of Eye (in
metres)
= 0.97√Height of Eye (in feet)
The above includes the effects of the curvature of the
earth’s surface and atmospheric refraction.
If a sextant angle is taken using an artificial
horizon then DIP does NOT have to be applied, since the artificial horizon
itself is the sensible horizon.
The altitude obtained after applying corrections for
index error and dip is also referred to as apparent altitude.
More on
Refraction
A ray of light arriving from a heavenly body also is
refracted when passing through the atmosphere. But the observer sees the star
at a position and sees no bending of the light, the position of the star
however as observed by the observer and the actual position differ; the star
appears higher in the sky due to refraction.
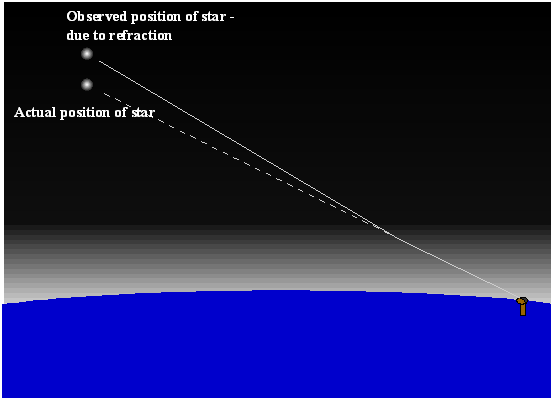
Atmospheric standard refraction, R0, is 0’ at 90°
altitude and increases progressively to approx. 34’ as the apparent altitude
approaches 0°:
‘Dip’,
‘refraction’, ‘semi‑diameter’ and ‘parallax’, and their causes
Calculations of celestial navigation refer to the
altitude with respect to the earth’s center and the celestial horizon.
But when taking observations the observer is on the
surface of the earth.
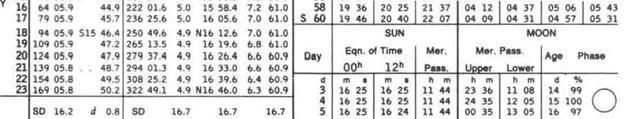
Parallax in altitude, is the difference in the in the altitude
as measured with respect to the sensible horizon and that with the rational
horizon. For distant objects it is not so significant since the distance is
huge and the angular difference is not much.
This parallax is of importance as a body is closer to
the earth like the Moon and the Sun, and to some extent for the planets, it
becomes progressively less for distant objects.

It decreases with growing distance between object and
earth and is too small to be measured when observing stars. Since the height of
eye is several magnitudes smaller than the radius of the earth, the observed
parallax refers to the sensible.
When observing sun or moon with a sextant, it is not
possible to locate the center of the body with sufficient accuracy. It is
therefore common practice to measure the altitude of the upper or lower limb of
the body and add or subtract the apparent semi diameter, SD, the angular
distance of the respective limb from the center.
The geocentric semi diameters of sun and moon are
given on the daily pages of the Nautical Almanac

The SD of the sun is given once for every 3 days –
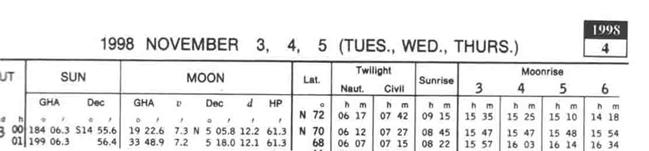
November 3, 4 5 whereas the SD of the Moon is given for each individual day. In
this case for all three days the SD is the same.